Are you curious to know what is the mean value theorem? You have come to the right place as I am going to tell you everything about the mean value theorem in a very simple explanation. Without further discussion let’s begin to know what is the mean value theorem?
In the realm of calculus, the Mean Value Theorem (MVT) stands as a fundamental concept that connects the instantaneous and average rates of change of a function. This mathematical principle, with its practical applications, holds significance in understanding the behavior of functions within a given interval. Let’s explore the Mean Value Theorem and its applications in detail.
What Is The Mean Value Theorem?
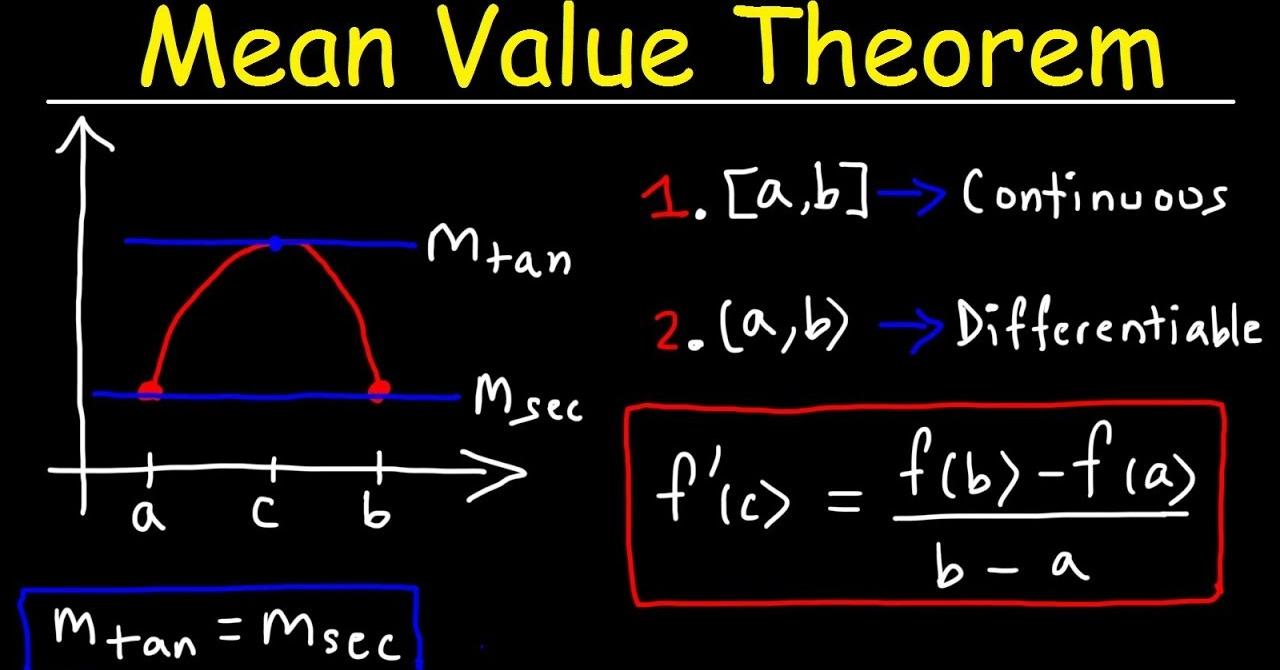
The Mean Value Theorem is a fundamental concept in calculus that applies to a differentiable function over a closed interval. Simply put, if a function is continuous and differentiable over a given interval [a, b], then there exists at least one point ‘c’ within that interval where the instantaneous rate of change (derivative) equals the average rate of change.
Understanding The Geometric Mean (Altitude) Theorem:
To comprehend the Mean Value Theorem, it’s helpful to explore related geometric concepts. The Geometric Mean (Altitude) Theorem involves the relationship between a right triangle’s legs and altitude. Understanding this theorem aids in grasping the concepts associated with the Mean Value Theorem.
Use The Geometric Mean (Leg) Theorem. What Is The Value Of A?
The Geometric Mean (Leg) Theorem is another geometrical concept that ties into the Mean Value Theorem. It deals with the relationship between the legs of a right triangle. When applied, it helps determine the value of ‘a’ in the context of the Mean Value Theorem.
Key Components Of The Mean Value Theorem:
- Continuity: The function must be continuous over the closed interval [a, b].
- Differentiability: The function must be differentiable over the open interval (a, b).
- Instantaneous and Average Rates: At some point ‘c,’ the instantaneous rate of change (derivative) equals the average rate of change over the interval.
Get Information About Advantages On Mainadvantages.
What Is The Mean Value Theorem Used For?
The Mean Value Theorem serves several practical purposes in calculus and real-world applications:
- Understanding Rates of Change: It provides insights into the relationship between instantaneous and average rates of change.
- Optimization: The theorem aids in solving optimization problems by identifying critical points where the derivative is zero.
- Physics and Motion: In physics, the Mean Value Theorem is applied to study motion, velocity, and acceleration.
Use The Geometric Mean (Altitude) Theorem. What Is The Value Of M?
Applying the Geometric Mean (Altitude) Theorem in the context of the Mean Value Theorem helps determine the value of ‘M.’ This step is essential in solving specific problems that involve geometric interpretations of calculus concepts.
Conclusion:
The Mean Value Theorem serves as a bridge between the abstract world of calculus and practical applications in various fields. Understanding its principles, along with related geometric theorems, enhances not only mathematical comprehension but also the ability to apply these concepts to solve real-world problems. As you navigate the intricacies of calculus, the Mean Value Theorem stands as a cornerstone, offering valuable insights into the behavior of functions within defined intervals.
FAQ
Which Is The Mean Value Theorem?
The Mean Value Theorem states that if a function f is continuous on the closed interval [a,b] and differentiable on the open interval (a,b), then there exists a point c in the interval (a,b) such that f'(c) is equal to the function’s average rate of change over [a,b].
What Is The Mean Value Theorem In Mit?
The reason it’s called the “mean value theorem” is because the word “mean” is the same as the word “average”. In math symbols, it says: f(b) − f(a) = f�(c) (for some c, a<c<b) b − a Provided that f is differentiable on a<x<b, and continuous on a ≤ x ≤ b.
What Is The Mean Value Theorem In Calculus For Dummies?
Now, imagine that you take a drive and average 50 miles per hour. The mean value theorem guarantees that you are going exactly 50 mph for at least one moment during your drive. Think about it. Your average speed can’t be 50 mph if you go slower than 50 the whole way or if you go faster than 50 the whole way.
What Is The Mean Value Theorem Conclusion?
The conclusion is that there exists a point in the interval such that the tangent at the point c , f c is parallel to the line that passes through the points a , f a and b , f b .
I Have Covered All The Following Queries And Topics In The Above Article
Use The Geometric Mean (Altitude) Theorem. What Is The Value Of M?
Use The Geometric Mean (Leg) Theorem. What Is The Value Of A?
What Is The Mean Value Theorem Used For
Use The Geometric Mean (Altitude) Theorem. What Is The Value Of M
What Is The Mean Value Theorem